Project highlights:

Optimal design, optimal control and parameter estimation of systems governed by partial differential equations (PDE) give rise to a class of problems referred to as PDE-constrained optimization (OPT-PDE). OPT-PDE pursues the idea to influence phenomena and processes, governed by PDEs. As PDEs describe almost every aspect of physics, chemistry, engineering, biology, finance etc., that fit into a continuum framework, OPT-PDE can be regarded as the ultimate/farthermost goal of any application problem to steer the underlying systems in a desired way.

As a starting point, we consider the OPT-PDE setting with a stationary or time-dependent reaction-diffusion equation as constraint, combined with pointwise (box) constraints of the state and the control variable, which latter is in addition sparse (localized).

The aim of the project is two-fold. On the modelling and discretization side we will

• study appropriate formulations of the cost functional and regularization techniques;
• by proper stabilization handle constraints and problem features applied on subregions of the control domain, not aligned with any discretization mesh;
• analyse the interplay between regularization, stabilization and discretization parameters in order to formalize their mutual dependence and how to balance the corresponding errors, the ill-conditioning of the arising algebraic systems of equations and the attainable accuracy of the computed optimal solution.

On the solution method side we will

• develop efficient preconditioners to speed up the linear solvers, used in the nonlinear solution procedure, inevitably required for the target OPT-PDE problem;
• develop two- and multilevel mixed nonlinear-linear methods to reduce the computational cost of the nonlinear solver on the finest discretization level, required to guarantee a desired level of accuracy;
• on all stages of development and implementation of the solution procedures and algorithms, enhance their performance on HPC resources.

First results:

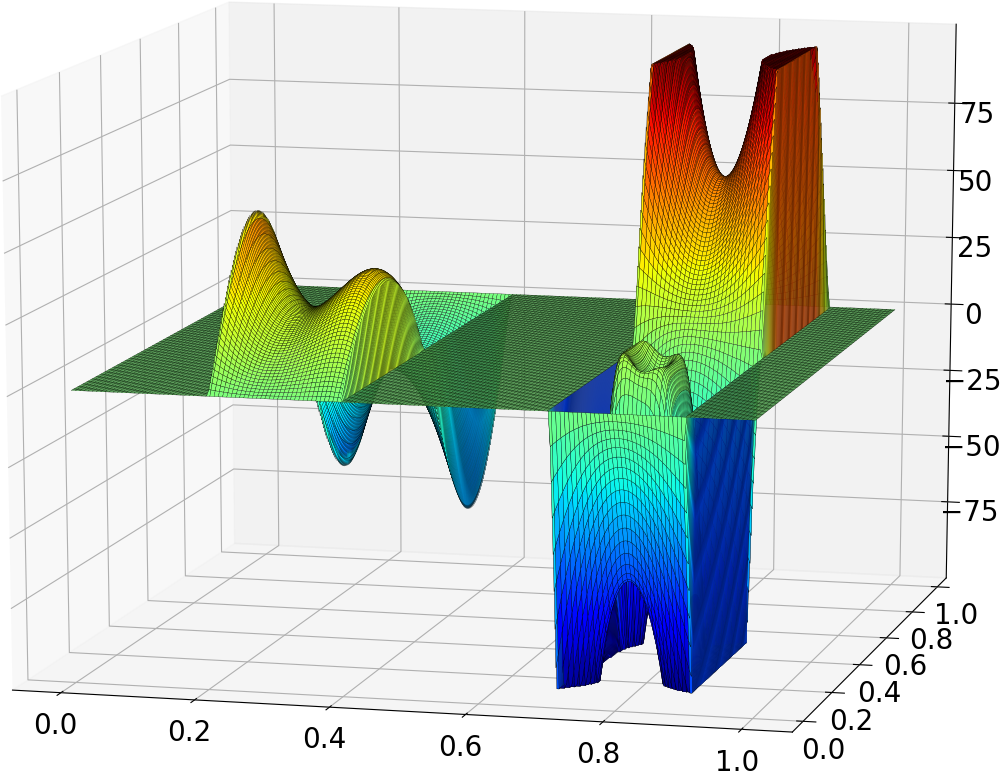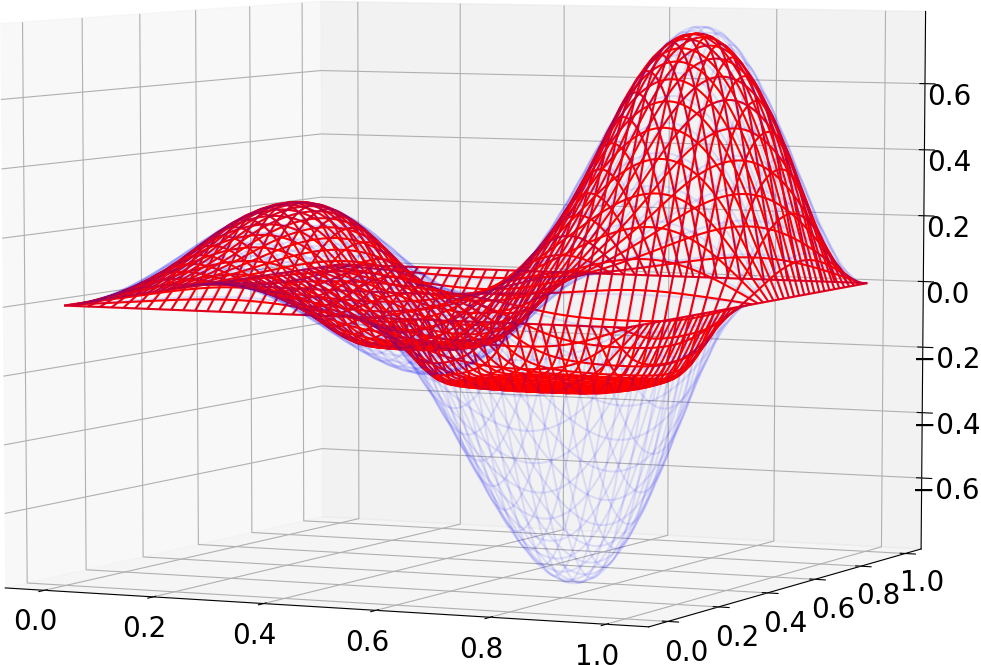
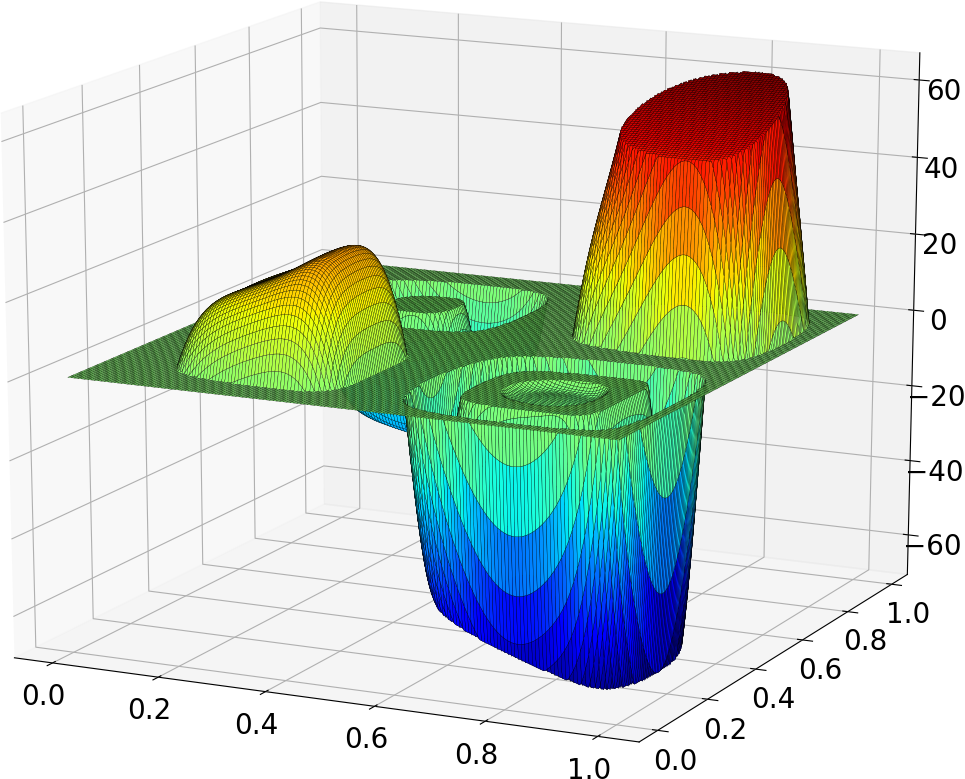
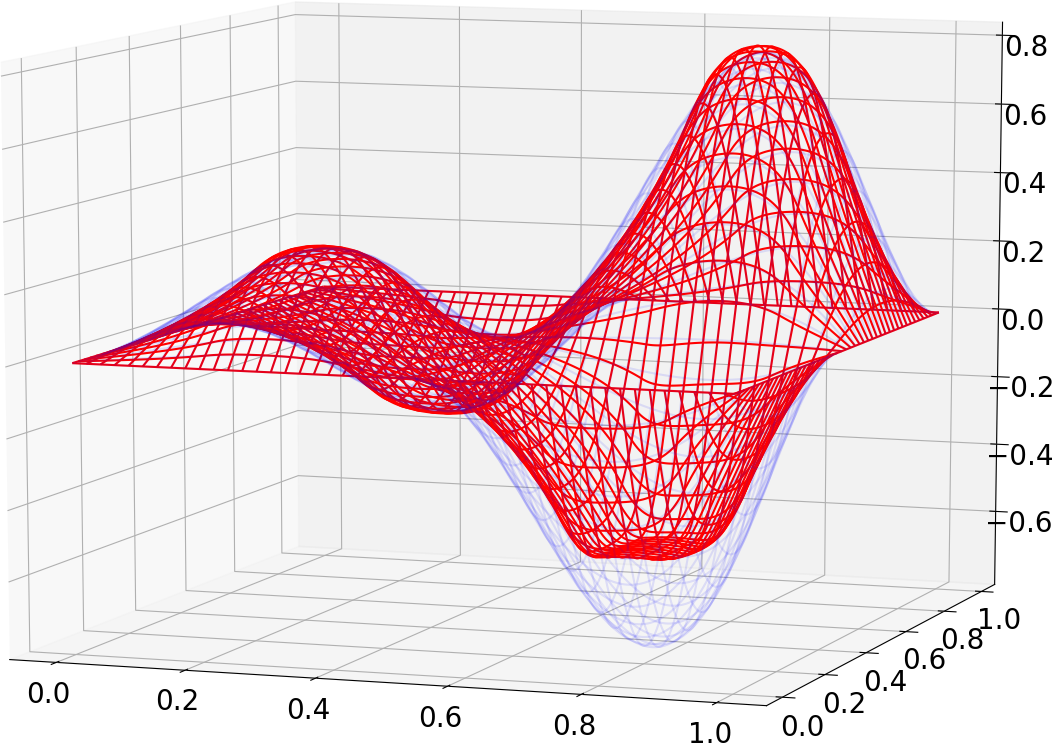
State	Control	State	Sparse control

Article in review: I. Dravins, M. Neytcheva, PDE-Constrained Optimization: Matrix Structures and Preconditioners