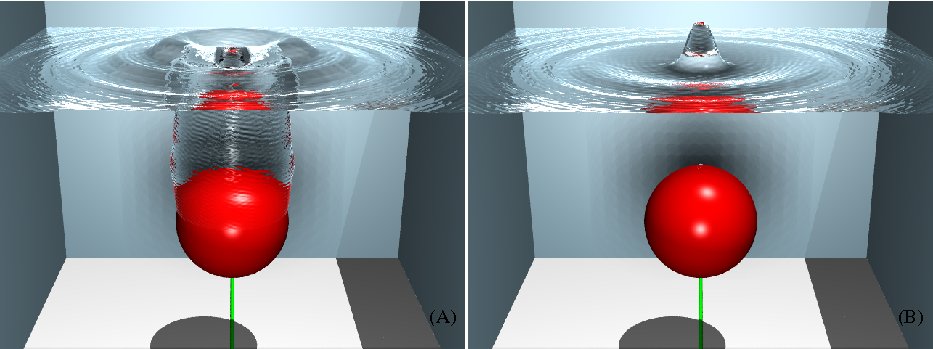

Due to its high impact on numerous industrial applications of vital importance today, pattern formation in multiphase media is among the key phenomena to model and simulate numerically. Multiphase processes advance through free contact surfaces (sharp fronts) to be accurately tracked by the numerical (discretization) methods.
We consider numerical simulations of mathematical models of morphological pattern formation and interface motion of multiphase microstructures, and in particular multiphase flow, based on the Phase-Field model. The aim of this project is to enable fast and reliable numerical solution of the large scale problems as arising from finite element discretizations of the above models. To this end we will consider the construction, analysis and implementation of a fully robust preconditioned iterative solution method based on local features of the Finite Element method discretization techniques. The preconditining method will target nonselfadjoint algebraic systems of equations as arising in dynamical multiphase microstructure simulations but will be applicable to a broader class of problems, arising from partial differential equations, discretized by the finite element method.
The developed solution techniques within this project are of general nature and could be successfully applied to problems arising in different application areas, such as Geophysics, namely, modelling of viscoelastic materials, modelling of glaciation/deglaciation cycles, including multiscale effects related to the contact area between glacier and Earth surface.
Publications:
Published in scientific journals:
[1] M. Do-Quang, G. Amberg,
The splash of a ball hitting a liquid surface: Numerical simulation of the influence of wetting, Journal Physic of Fluid, 2008.
[2] Petia Boyanova, Svetozar Margenov, Maya Neytcheva,
Robust AMLI Methods for Parabolic Crouzeix-Raviart FEM Systems,
Editors: A. Havasi, I. Farago, S. Margenov, Z. Zlatev. Special Issue
on Advanced Computational Algorithms,
Journal of Computational and Applied Mathematics (JCAM),
235 (2010), 2010, 380-390.
[3] Maya Neytcheva,
On element-by-element Schur complement approximations,
Linear Algebra and Its Applications, in press.
Available online 1 May 2010
[4] O. Axelsson, R. Blaheta, M. Neytcheva.
Preconditioning for boundary value problems
using elementwise Schur complements.
SIAM Journal on Matrix Analysis and Applications, 31 (2009), 767-789.
[5] Carlson, A., Do-Quang, M. and Amberg, G.
Droplet dynamics in bifurcating channels.
International Journal of Multiphase Flow, 36, p397, (2010).
[6] Do-Quang, M. and Amberg, G.
Numerical simulation of the coupling problem of a solid sphere impacting on a liquid
free surface.
Mathematics and Computers in Simulation, 80, p.1664 (2010).
[7] Do-Quang, M. and Geyl, L. and Stemme, G. and van der Wijngaart, W. and Amberg, G.
Fluid dynamic behavior of dispensing small droplets through a thin liquid film.
Journal Microfluidics and Nanofluidics, 9, 303, (2010).
[8] Carlson, A., Do-Quang, M. and Amberg, G.
Modeling of dynamic wetting far from equilibrium,
Journal Physics of Fluids, 21, p121701. (2009).
Published in Conference Proceedings:
[1] M. Neytcheva, M. Do-Quang, He Xin,
Element-by-element Schur complement approximations for general nonsymmetric
matrices of block two-by-two form. To appear in
Springer Lecture Notes in Computer Science (LNCS).
[2] Do-Quang, M., Carlson, A. and Amberg, G.
Modeling of Drops Impact and Penetration into Porous Substrate.
Proceedings of International Conference on Multiscale Complex Fluid
Flows and Interfacial Phenomena, Brussels 7-10 Nov. (2010)
[3] Carlson, A., Do-Quang, M. and Amberg, G. Dissipation in rapid dynamic wetting.
Proceedings of International Conference on Multiscale Complex Fluid Flows and Interfacial
Phenomena, Brussels 7-10 Nov. (2010)
[4] Do-Quang, M., Lundell F., Oko, A., Swerin, A. and Amberg, G. Droplet Impact and
Penetration on a Porous Substrate: a Phase Field model Proceedings of the 23rd Nordic Seminar
on Computational Mechanics, Stockholm 21-22 Oct., p.291, (2010)
[5] Do-Quang, M., Carlson, A. and Amberg, G. The impact of ink-jet droplets on a paper-like
structure. Proceedings of 7th International Conference on Multiphase Flow, Tampa, May 30 –
June 4, p.224, (2010).
[6] Carlson, A., Do-Quang, M. and Amberg, G. Characterization of droplet dynamics in a
bifurcating channel. Proceedings of 7th International Conference on Multiphase Flow, Tampa,
May 30 - June 4, p. 532, (2010).
[7] Carlson, A., Do-Quang, M. and Amberg, G Spontaneously spreading liquid droplets in a
dynamic wetting process. Proceedings of the EUROTHERM-84, Namur, Belgium, May 24-27.
(2009).
Technical reports and manuscripts in progress:
[1] Owe Axelsson and Maya Neytcheva.
A General Approach to Analyse Preconditioners for Two-by-Two Block Matrices
TR 2010-029, Institute for Information Technology, Uppsala University,
November 2010. Submitted.
[2] Xin He, Maya Neytcheva, and Stefano Serra Capizzano.
On an Augmented Lagrangian-Based Preconditioning of Oseen Type Problems
TR 2010-026, Institute for Information Technology, Uppsala University,
November 2010. Submitted.
[3] M. Neytcheva, E. Bšngtsson, E. Linner.
Finite-element based sparse approximate inverses for block-factorized preconditioners. TR 2010-010, Institute for Information Technology, Uppsala University, March 2010.
Submitted to the special issue on "Numerical and Applied Linear Algebra" of the journal
Advances in Computational Mathematics.
[4] Do-Quang M., Engblom, S., Tornberg, A-K. and Amberg, G. “Phase field method for
interfacial fluid flow with soluble surfactant In 63rd Annual Meeting of the APS
Division of
Fluid Dynamics, Long beach, USA, 21-23 Nov. (2010).
[5] Khan, M., Do-Quang, M., Amberg, G., Wu, J. and Aidun, C. Bulk Rheology of Noncolloidal
Deformable Fiber Suspension. In 63rd Annual Meeting of the APS Division of Fluid Dynamics,
Long beach, USA, 21-23 Nov. (2010).
[6] Carlson, A., Do-Quang, M. and Amberg, G. Modeling of dynamic wetting far from equilibrium
In 62nd Annual Meeting of the APS Division of Fluid Dynamics,
Minneapolis, USA, 22-24 Nov. (2010).
[7] Do-Quang, M., Carlson, A. and Amberg, G. High performance computing of free surface Phase
Field simulations. In European Conference on Numerical Mathematics and Advanced Applications
(Enumath), Uppsala, Sweden, (2009).
[8] A. Carlson; M. Do-Quang; G. Amberg.
Dissipation in rapid dynamic wetting
submitted to Journal of Fluid Mechanics
[9] P. Boyanova, Minh Do-Quang, M. Neytcheva.
Non-conforming FEM for the Cahn-Hilliard Equation
Manuscript in progress, 2010.
[10] Petia Boyanova, Minh Do-Quang, Maya Neytcheva,
Contribution from LSSC 2011, Sozopol, Bulgaria (to be submitted)
[11] M. Do-Quang, S.Engblom.
On modeling and simulation of surfactants in diffuse interface flow,
Manuscript in progress, 2010.